Computer Graphics Forum 33, 8, 167–176. 2014.

Higher Order Ray Marching

¹ Universidad de Zaragoza, I3A

Render of participating media with equiangular sampling and several numerical solvers. Top row: Rendered image. Bottom row: Absolute error vs. ground truth in false color (blue is lowest, red is highest). (a) Standard ray marching, rectangle quadrature rule (17s). The image shows banding at the cone of light. (b) Monte-Carlo integration (19s). The image shows high frequency noise. (c) Bogacki-Sampine method: an order three embedded (adaptive) initial value problem solver (1m 15s). (d) Nested Simpson quadrature rule: an order two nested (adaptive) quadrature rule (17s). (e) Monte-Carlo reference (5h).

Abstract

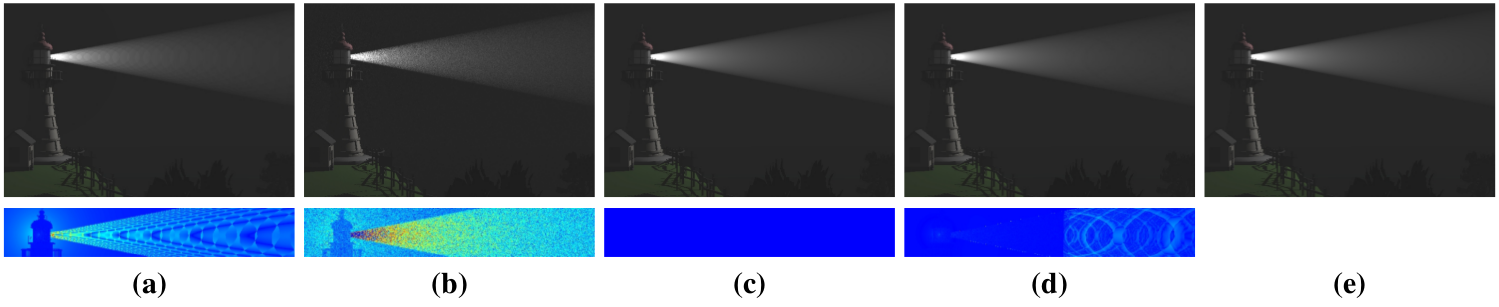
Rendering participating media is still a challenging and time consuming task. In such media light interacts at every differential point of its path. Several rendering algorithms are based on ray marching: dividing the path of light into segments and calculating interactions at each of them. In this work, we revisit and analyze ray marching both as a quadrature integrator and as an initial value problem solver, and apply higher-order adaptive solvers that ensure several interesting properties, such as faster convergence, adaptiveness to the mathematical definition of light transport and robustness to singularities. We compare several numerical methods, including standard ray marching and Monte Carlo integration, and illustrate the benefits of different solvers for a variety of scenes. Any participating media rendering algorithm that is based on ray marching may benefit from the application of our approach by reducing the number of needed samples (and therefore, rendering time) and increasing accuracy.

Files

Source code

Parts of the source code for this paper have been released to the public domain.

qdt - A C++11 header-based library for quadrature integrators.

BibTeX

Acknowledgements

We would like to thank Adrian Jarabo for his constructive criticism about this work. This research has been partially funded by the European Commission, 7th Framework Program, through projects GOLEM, VERVE, the Spanish Ministry of Science and Technology, project TAMA and by the Gobierno de Aragón (project CTPP6/11).